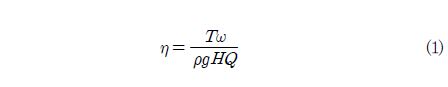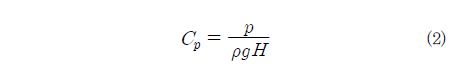Effect of Guide Nozzle Shape on the Performance Improvement of a Very Low Head Cross Flow Turbine

2.1. Cross Flow Turbine Model

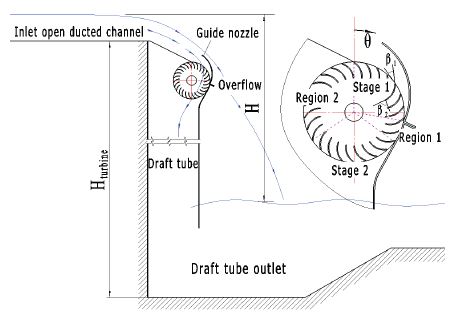
Figure 1 shows the schematic view of the new type of very low head cross flow turbine model. The structure of the turbine consists of an open ducted water channel, runner and draft tube, without guide vane and nozzle. The draft tube is included in the turbine to reduce the pressure at the top of the draft tube, thereby sucking water into the turbine chamber. The water flow from open water channel separates into two flows: one part of the water flows into the draft tube through the runner passage, and the other overflows down the river with some materials of sediment, preventing damage to the runner structure.

The design point of the present test turbine for the effective head is H=4.3m, which is very low in contrast to other typical cases of cross flow turbine’s head. More details for design parameters are shown in Table 1.

To improve the performance of turbine, a guide nozzle is attached at the inlet of turbine as shown in Fig. 2. Table 2 shows more detail parameters of the different turbine guide nozzle radius. To investigate the effect of the guide nozzle radius on the performance, a series of cases were studied from Cases A to E, with nozzle opening increasing as radius of guide nozzle increases. To further improve the turbine performance, the case (Case B) with maximum efficiency is selected for the second step. In this process, the radius is kept constant and the nozzle angle (θ_Nozzle) is changed to investigate the effect of guide nozzle length on the turbine performance as shown in Fig. 2 and Table 3. Case S has the same radius (R) and nozzle angle (θ_Nozzle) with Case B1. The only difference is that the guide nozzle shape for Case S has been modified by making a smoother inlet as seen in Fig. 2. The outside guide nozzle shape is simplified for better modeling and mesh quality. The outside guide nozzle shape has no influence on the performance of the turbine.

Fig. 1

Schematic view of the very low head cross flow turbine

Table 1

Design parameters of turbine

Fig. 2

Variation of the turbine models with guide nozzle

Table 2

Cases of the different turbine guide nozzle radius

2.2. Numerical Methods

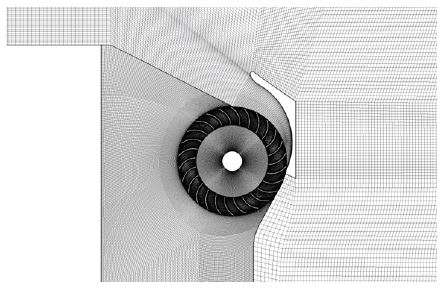
Computational Fluid Dynamic (CFD) analysis is a very useful tool for predicting hydraulic machinery performance at various operating conditions[10]. In this study, a commercial CFD code of ANSYS CFX [11] is employed as a solver. The numerical grids are made in two dimensional geometry in order to shorten the calculation time. Gravity is included for two phase transient calculation and SST turbulence model is adopted. The grid constitutes greater than 4.5×10⁵ elements for the whole flow field. The boundary condition of normal speed is set for the water flow at the inlet and outlet, and the velocity of 0 m/s is set for the air flow. The boundary condition of opening is set at the open duct and down river domain. The boundary conditionals are shown in Table 4.

Figure 3 shows the fine hexahedral numerical grids of the flow field including cross flow turbine model. The area of the runner passage can be divided into four regions by the flow patterns in the runner passage as shown in Fig. 1. Stage 1 gives first output power and Stage 2 gives second output power. However, Regions 1 and 2 consume output power by hydraulic loss.

Fig. 3

Fine hexahedral numerical grids of the flow field (Case S)

Table 3

Cases of the different guide nozzle angle θNozzle

Table 4

Numerical methods and boundary setting

3.1. Performance curves

For this very low head cross flow turbine, the efficiency is calculated based on the potential energy difference between the upstream and downstream locations of the turbine model by the following equation:

where η is the efficiency of the turbine; T is the output torque; ω is the angular velocity; H is the water level difference between the upstream and downstream locations of the turbine model; and Q is the water flow passing through the draft tube.

Fig. 4

Performance curves by turbine guide nozzle radius R

Fig. 5

Performance curves by turbine nozzle angle θNozzle

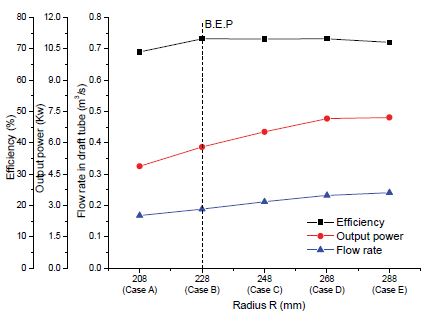
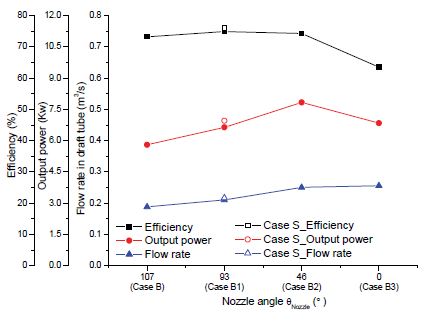
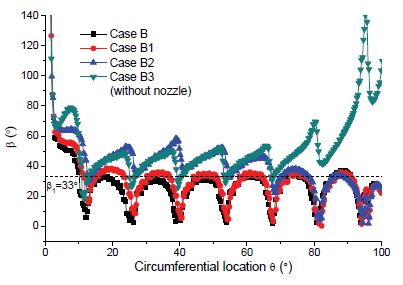
Figures 4 and 5 show the performance curves by the turbine guide nozzle radius and nozzle angle θ_Nozzle, respectively. Larger guide nozzle radius is good for guiding the flow into the draft tube, and contributes to generating more output power. The efficiency of the turbine reaches maximum at Case B for which the radius of guide nozzle is R=228mm. From Fig. 5, it is inferred that the flow rate in the draft tube increases with shortening of the guide nozzle length. Especially for Case B3 without nozzle, the flow rate in draft tube is the maximum in comparison with the series of cases for different nozzle angle. The maximum efficiency reaches at Case B1 with 93° nozzle angle. The efficiency reduces rapidly without guide nozzle. Comparing Case B1 and Case S, the guide nozzle with smooth inlet shape (Case S) further improves the efficiency of the turbine.

3.2. Output power distribution

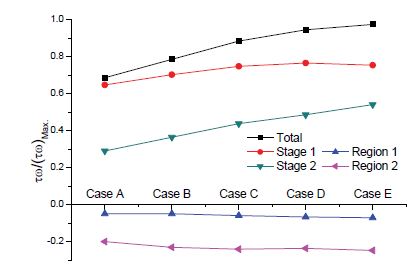
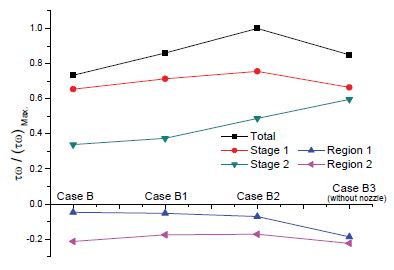
In order to investigate the output power of the very low head cross flow turbine with open duct, the output power on the circumferential is divided into four regions and getting the local output power ratio as shown in Figs 6 and 7. The values of output power at every region are divided by the maximum one.

Figure 6 shows the local output power ratio at each region by guide nozzle radius (R). The Case E has the maximum output power, which means the output power ratio of Case E is 1 as shown in Fig. 6. The larger the guide nozzle radius (R), the more flow rate is sucked into the draft tube through the runner passage. It can be also observed that the influence of increasing the guide nozzle radius (R) obviously rises to output power at Stages 1 and 2. However, the influence on the Regions 1 and 2 is poor, where loss of torque occurs.

Figure 7 presents the local output power ratio at each region by nozzle angle θ_Nozzle. From Cases B to B3, with the nozzle angle (θ_Nozzle) reducing, the output power at Stages 1 and 2 increases. The total output power reaches the maximum one at Case B2. However, for Case B3 without guide nozzle, the output power at Stage 1 reduces, while that at Stage 2 rises. Moreover, the negative torque rise rapidly at Regions 1 and 2, so the torque loss increases more in the case without guide nozzle at these two regions. The guide nozzle suppress the negative torque at Regions 1 and 2 effectively. Nevertheless, the efficiency and output power reduces if the nozzle length is too long.

In order to examine the influence factors for the performance variation of the cross flow turbine models in detail, internal flow is investigated.

Fig. 6

Averaged local output power ratio at each region by guide nozzle radius (R)

Fig. 7

Averaged local output power ratio at each region by nozzle angle (θNozzle)

Fig. 8

Schematic of velocity triangle at blade entry and exit

3.3. Velocity triangle components distribution

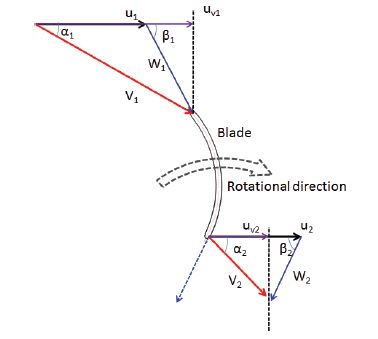
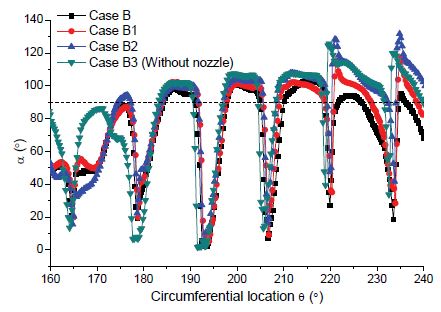
For turbomachinery, the velocity triangle distribution on the entrance and exit of the blades is a very important factor on the performance[12]. The velocity triangle components on the turbine runner blades entry and exit are plotted to investigate the influence of guide nozzle on the performance. Figure 8 shows the schematic of velocity triangle at blade entry and exit. α is the absolute angle that is between absolute velocity(V) and tip rotational speed(u). β is the relative angle that is between relative velocity(w) and tip rotational velocity(u). β₁ is the inlet angle of blade. For maximum efficiency point, the relative angle(β) should be close to the inlet angle of blade(β₁), and the absolute angle(α) of outflow at Stage 2 should be close to 90°[13].

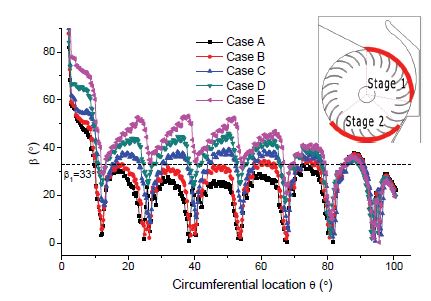
Figure 9 shows the relative angle(β) distribution at the runner blade passage inlet periphery of Stage 1 by guide nozzle radius(R). The dashed line in the figure is the inlet angle of blade β₁=33°. There are larger variations on the β along with the circumferential location because of the blade. The β on the runner passage part at Stage 1 shows that Case B is the one closest to β₁, which means Case B has the least energy loss by flow through the runner passage at Stage 1. A larger guide nozzle radius causes higher β, likewise, a smaller guide nozzle radius causes lower β, when compared to β₁. Guide nozzle radius gives significant effect on the relative angle at the entry of Stage 1.

Figure 10 shows the relative angle(β) distribution at the runner blade passage inlet periphery of Stage 1 by nozzle angle(θ_Nozzle). An appropriate nozzle angle for the guide nozzle plays an important role on the relatively angle(β). From Fig. 10, the β of Case B1 is closer to β₁ than that of Case B, and with the nozzle angle(θ_Nozzle) more shortened, the β is farther away β2. That’s why the efficiency curve shows that Case B1 has the maximum one(See in Fig. 5).

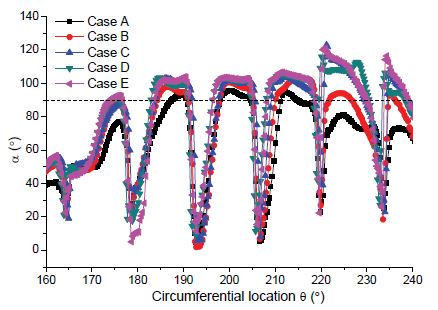
Figures 11 and 12 shows the absolute angle(α) distribution at the runner blade passage outlet periphery of Stage 2 by guide nozzle radius (R) and nozzle angle (θ_Nozzle), respectively. The absolute angle (α) shows that the smaller guide nozzle radius and larger guide nozzle shape are better for the absolute angle being nearer to 90° at the outlet of Stage 2. The turbine generates most of its output power from circumferential location of 180° to 220°.

An appropriate guide nozzle radius and nozzle angle plays an important role on the velocity triangle distribution. By increasing the guide nozzle radius and shortening the guide nozzle shape results in an increased relative angle at Stage 1, however, the absolute angle at Stage 2 moves slightly farther away from 90°.

Fig. 9

The relative angle(β) distribution at periphery of Stage 1 by guide nozzle radius (R)

Fig. 10

The relative angle(β) distribution at periphery of Stage 1 by nozzle angle (θNozzle)

Fig. 11

The absolute angle(α) distribution at periphery of Stage 2 by guide nozzle radius (R)

Fig. 12

The absolute angle(α) distribution at periphery of Stage 2 by nozzle angle (θNozzle)

Fig. 13

Pressure contours

Fig. 14

Pressure distribution on the local blade surface

Fig. 15

Velocity vector at the nozzle passage

Fig. 16

Pressure distribution on the inner surface of guide nozzle

3.4. Effect of turbine guide nozzle

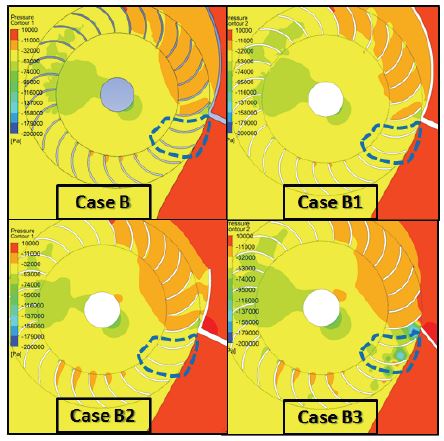
The effect of the guide nozzle on the turbine performance by the nozzle installation is investigated. From Figs. 5 and 7, it can been seen that for the turbine without guide nozzle, the efficiency and output power drop rapidly and also the negative torque rises considerably at Region 1. The pressure contours around the runner are examined as shown in Fig. 13. It can be observed that larger pressure difference at Region 1 of Case B3, but with guide nozzle (Cases B, B1 and B2) the pressure difference relatively distributes evenly. In order to investigate the guide nozzle effect, the averaged pressure distribution on the local blade surface at Region 1 are plotted quantitatively as shown in Fig. 14. The pressure is averaged from two blades surface pressures at Region 1 (Fig. 13), and normalized to C_p as in the following equation:

where p is the averaged pressure on two blades surface at Region 1. The pressure around the blade shows the pressure of suction side is larger than that of pressure side, which means the torque generated by pressure difference is negative. This figure also shows the drastic change in pressure difference between the cases with and without nozzle. Cases B to B2 show similar pressure difference. It is conjectured that this change affects the torque value at Region 1.

3.5. Effect of guide nozzle inlet shape

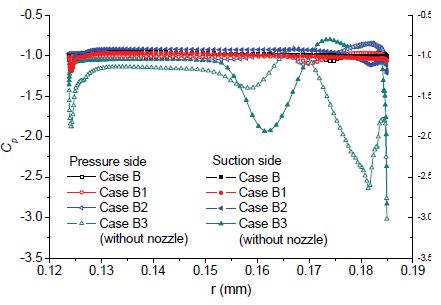
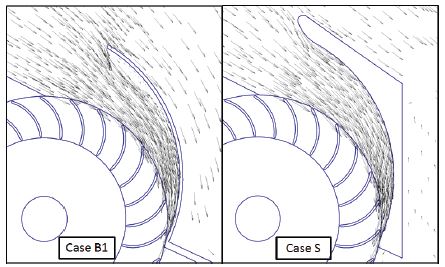
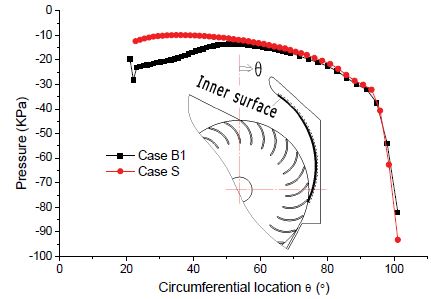
Only the inlet shape of guide nozzle is different between Cases B1 and S. Fig. 15 shows the velocity vector at the nozzle passage for both at Cases B1 and S. There is a region of flow separation flow near the inlet of the guide nozzle in Case B1. However, the separation flow disappears in Case S with smooth shape of guide nozzle inlet. The pressure distribution on the inner surface of the guide nozzle is plotted for Cases B1 and B as shown in Fig. 16. It can been seen that the pressure of Case S on the inner surface of guide nozzle changes gradually. However, for the Case B1, there are large variations of pressure at the inlet of guide nozzle, and the pressure values of these two cases remains same after the circumferential location of about 45°. It is conjectured that the separation flow at the guide nozzle inlet causes the decrease of flow rate, and leads to a drop of efficiency as shown in Fig. 5.